
#Principle of inclusion and exclusion how to#
I dont want to know the answer, I just want to know how to proceed. In combinatorics, the inclusionexclusion principle (also known as the sieve principle) is an equation relating thesizes of two sets and their union. How do I move ahead or where did I go wrong? Or $$25 = |A| + |B| + |C| - |A ∩ B| - |A ∩ C| - |B ∩ C|$$īut now I am stuck because the statement above does not give me any information about students who answered exactly 1 question. $$50 = |A| + |B| + |C| - |A ∩ B| - |A ∩ C| - |B ∩ C| + 25$$ The principle of inclusion-exclusion is an important result of combinatorial calculus which finds applications in various fields, from Number Theory to Probability, Measurement Theory and others. The principle of Inclusion-Exclusion states that: It is important to notice that since any two of given sets can have a non-empty intersection, the. $|A \cap B \cap C| = 25$ (Students who answered all $3$) The principle gives a direct formula for computing S n.$|A \cup B \cap C^c)| = 10$ (Students who did not answer $Q_3$).$|A \cup C \cap B^c| = 14$ (Students who did not answer $Q_2$).

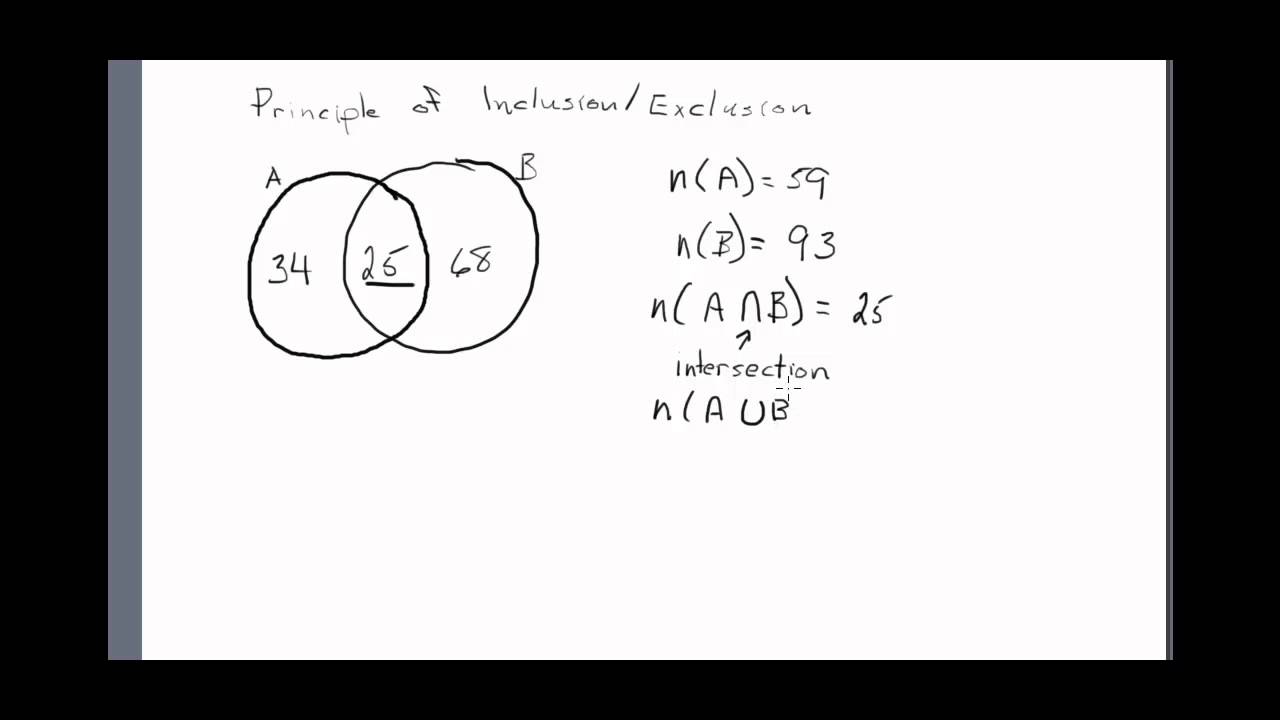
$|B \cup C \cap A^c| = 12$ (Students who did not answer $Q_1$).One uses inclusion-exclusion, but there is also another, slightly simpler, solution. If $12$ students did not answer $Q_1$, $14$ did not answer $Q_2$, $10$ did not answer $Q_3$ and $25$ answered all $3$ questions, then how many students answered exactly $1$ question? (b)How many ways are there to form a study group that contains at least one of Bob, Sue, and Alicia There are several ways to approach this problem. Web Inclusion/exclusion principle for belief functions. All the students answer at least $1$ question. The Inclusion Exclusion Principle and Its More General Version. There are $50$ students in a class who are given a test with $3$ questions on it: $Q_1$, $Q_2$, and $Q_3$. I am trying to solve the following question:


 0 kommentar(er)
0 kommentar(er)
